Opportunities gained......or lost

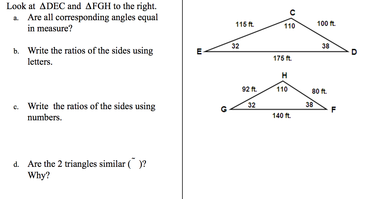
Figure 1

Recently we started a lesson on similar triangles.  I thought a lot about what to have the kids do, and a small part of me (very small) wanted to 'direct teach' the lesson to save time - we are behind, after all, and if I just told them what similar triangles were and what to do with them we could move on to the next lesson.  In the past, I would typically give them scripted notes that had a set of similar triangles, such as the example in figure 1.  I would explain what similar triangles were, and how to find different things about them, such as angle measures  and/or side lengths.  The students would typically do well with this and then we could say that we had 'learned' similar triangles.  

---

This year I wanted to provide a richer opportunity for them.  I put up the following directions:

• Use your math resources to figure out what it means for triangles to be similar.
• Once you are comfortable with the notion of similar triangles, take the colored paper in the back and create a set of similar triangles.  You can use any other tools as well, such as rulers and/or protractors.
• After you create your set, be able to convince somebody that they are similar by using a mathematical argument

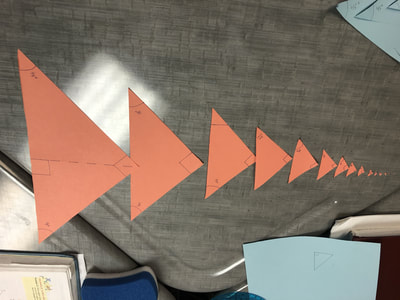
I purposely made the direction vague.  Some students asked me what similar triangles were, but I pushed it back to them to use their resources, such as their phone, computer, or textbook, to figure it out.  Some students also asked what I meant by a 'set' of similar triangles.  I asked them to look up that word as well and then decide what to do.  I had to laugh because some students just really needed me to tell them 'how many' triangles they needed to make.  I did not want to tell them because I did not want to give them a threshold at which to stop learning.
​
As time passed, many students were using their protractors to create two triangles that had the same angle measures.  Two seemed to be the most popular number of triangles.  There were a lot of struggles going on as measurements were being made and sides were being measured.  Some students used a lot of paper, while others used a sheet or two.

With about ten minutes to go in the block, two of my students, Filip and Haden, asked me to come and look at their set.  I walked over to their desk and this is what I saw:

Needless to say, I was floored!  The expectation in my head was to see two triangles that were created using straight edges and rulers.  I of course inquired about what had happened and what led them to this result, and I found out what actually happened: they used one sheet of paper and created thirteen (or so) triangles in about 5 minutes.  All of the triangles were similar, but the beauty of it was that they did it by folding the paper - rulers and protractors were not needed (one is shown in the picture, but it wasn't used).  The best part was that I was going to demo to the whole class how to 'quickly' create two similar triangles (by overlaying them on each other), and instead I had these two students demonstrate a much more powerful demonstration.

I was really thankful afterwards that I didn't give in to the urge to instruct directly because this opportunity would have been completely lost.  Instead, it was gained, and was a nice lead in for the next class, as I had Haden and Filip lead the class on how to construct so many similar triangles in such a short time.