 David Perkins spoke on the last day of the institute about how to take these ideas back to our schools. He mentioned the 5-year effect: new ideas get implemented, get traction, then slowly lose that energy and eventually die off. So, how do we make sure the energy does not fall away, and that key ideas and frameworks can have a long life? One thing that many schools do use is what Perkins calls the "installation model" of implementing change. Some training happens, maybe posters and brochures are made, teachers implement it, but then over time things begin to change back to the way they were. How do we combat this and ensure longevity? David talks about the "ecological model" of introducing change which has 4 main "legs":
Please check out the full article on "Giving Change Legs"
0 Comments
Wow! What a week! Things are beginning to wind down here in Cambridge and will culminate tomorrow when Tina Blythe and David Perkins, both of the Project Zero team, give a talk on "Giving Change Legs," which I believe will help us to utilize many of the awesome things we have learned here this week. First I included a few pictures. Then I include some discussion on the course "All Learners Learning Every Day."
All Learners Learning Every Day (ALL-ED) by Rhonda Bondie
Key takeaways:
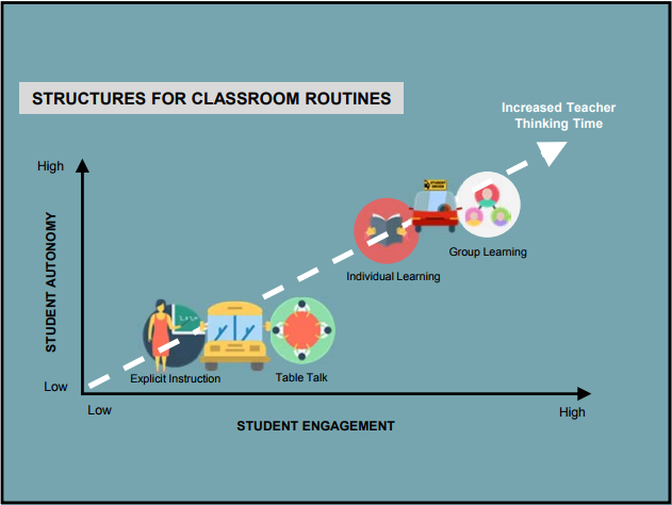
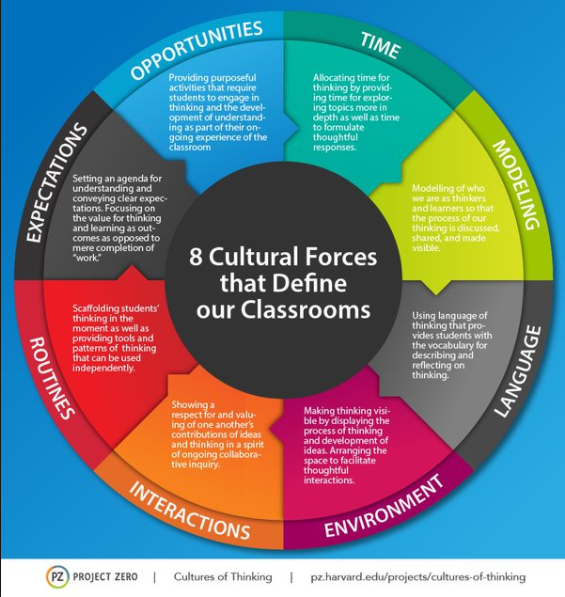
Creating a Culture of Thinking right from the start
Creating thinking opportunities from a mathematics perspective What is a color, symbol, and image that captures the Project Zero classroom thus far? Here is my take! Color: Yellow - I chose yellow because I think yellow represents energy, brightness, and excitement. These qualities have been on display here for two days!  Symbol: No spoon feeding - I believe that to use many of the great ideas presented here at project zero effectively, spoon feeding of students needs to be kept to a minimum. In math class, spoon feeding can take many forms, such as: showing them the recipe to do a math problem as opposed to the students creating the recipe; giving them the answers to a set of questions as opposed to them using other tools to gather the answers, such as Wolfram Alpha, Desmos, or each other; handing them a review sheet of problems that will be on the test as opposed to them coming up with the ideas and concepts that they will be tested on, to name a few.  Image: An awesome buffet - A great buffet, like the one shown at the right, presents a lot of appealing options. We have the freedom of choice to pick the items that we like the most, and also to leave what we won't eat. We also can go back to the buffet later, and pick items that we want again, or new items. This institute reminds me of that because we have been presented with a plethora of appealing ideas, many of which we want to take, and some that we may not be ready for. We have the freedom of choice to pick the ideas that we want to try to implement, and ones that we may want to come back to later. Slow LookingDefinition of Slow-looking: Taking time to notice more than meets the eye at first glance The themes of slow looking
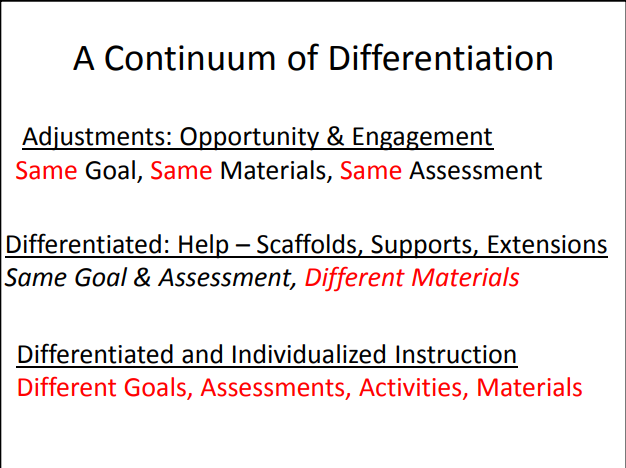
 I was thinking about the applications to math class, and at first was a bit puzzled as to what that would look like. After discussing it with my study group (STUDY GROUP O ROCKS!), the slow-looking does not have to involve an image, it could be a writing, a poem, an image, or work from a math problem. Many times in class, students will use a solution guide for a math problem, and after a minute or to proclaim, 'I have no idea what they are doing here!' It is in this instance that I think I will walk them through 'slow-looking' at the solution, and remind them of the quote to the left! The Teaching for Understanding (TfU) framework We assume we teach for understanding because we equate knowledge and understanding; knowledge is vital and critical, but just having a lot of knowledge doesn't make for understanding. What is it that a child will be able to do better if they understand? The goals of the Teaching for Understanding framework:  What does it mean to understand? Identify something that you understand really well. For me, it was finding solutions of a quadratic equation. My evidence is that I can find the solutions in multiple ways; I know what the solutions look like; all methods of solving result in the same solutions. What does understanding look like? 1. successful at something 2. know the pattern of doing things 3. you can teach it well 4. can analyze into component part and synthesize into whole 6. big picture in relation to details 7. anticipate 8. you can make connections 9. have a greater metacognition 10. can problem solve 11. can do it in a variety of ways What helps understanding develop? - doing multiple times - errors, mistakes, mishaps - mentor, guide, structure - explain it to someone - deconstructing - curiosity - exploring various ways to do something - observe - different perspectives - reflection - applying, implementing - try it again and again  What does teaching for understanding look like in the classroom? Some of the key questions from our study group today:
 To encapsulate day 1 of the Project Zero classroom, I am going to utilize the See-Think-Wonder thinking routine from "Making Thinking Visible" by Ron Ritchhart, Mark Church, and Karin Morrison. Below I highlight the steps of the routine and apply it to the first day... What do you SEE? Today I saw over 360+ educators from 30 countries. I saw an enthusiasm that would motivate any teacher. I saw people that I have read about: David Perkins, Howard Gardener, Mark Church, and Daniel Wilson. I saw a study group of people that I did not know at 9:00 this morning, but by 4:00 was working with them to create a visual arts representation of a poem that we were studying. I saw a campus with incredible architecture. I saw other educators that motivated me to want to be the best that I could be. I saw a thoughtful place that I want to be a part of for the next four days. I saw a place that I can't wait to tell my colleagues about. What did you HEAR (this technically is not part of the routine, but it was too important to pass up)? I heard many incredible things:
 What do you THINK is going on here? I think this is an institute that is a must for any educator at some point in their lives. I think I made a great choice in taking the time out of my summer to come here. I think this institute will help my students understand their content, think critically about it, and reflect thoughtfully about what they have learned. What does this make you WONDER? This makes me wonder why I didn't come to this institute sooner. It makes me wonder how my students learned anything in my early years of teaching. It makes me wonder why I thought I had teaching perfected when I was 25 but how at age 45 I realize I have so much more to learn. Some other pics from the day...
 Many times I have heard fellow teachers say things like, "if I could just teach and not grade", "my whole weekend was spent grading papers", or "I wish I could hire somebody to do all my grading for me!" It is true that stacks of papers are an inevitable part of teaching at any grade and any level, but as Ron Ritchhart states in his book Creating Cultures of Thinking: The Eight Forces We Must Master to Truly Transform Our Schools, "Taking a stack of papers home to read through and mark is rarely an energizing task for any teacher" (p. 94). Amen to that! Last year, I had four sections of one particular course. Every time I gave a test, I had about 120 tests to grade. If you figure about 15 minutes per test, that is 1800 minutes, which is 30 hours of grading, and that was per chapter. Furthermore, I teach six sections and this only accounted for four of the sections. What about the other two? I knew, and accepted, that grading would have to be done at home, but I simply didn't have time to do all the necessary grading and have the time to plan student-centered, collaborative, thought provoking lessons that I knew were so necessary for an effective math classroom.  So what was it going to be, grading or planning? Could it be both? One answer may lie in a re-allocation of time during the school day. Ritchhart mentions a teacher named Nathan that meets weekly with each of his grade 12 students to give feedback on their writing. Nathan sets up a meeting schedule and each student sits with him and they have a discussion about their paper. He gets to know his students better, is able to establish a rapport with them during these sessions, and is sure that the students receive the feedback (as compared to written feedback where Nathan wasn't sure if students read the feedback or not). When I read this, I thought 'Why couldn't I do this for math tests? Nathan did this for literature, why not Math?' I put this into action for the third unit test of the year. I built a schedule of 10-minute time slots using signupgenius.com, based on the days and times that I was available. For some of the days, I set up three time slots for the morning, two during lunch time, and three after school. Other days, I didn't have any in the morning, but scheduled three during lunch, two during my prep, and three after school. The time slot allotment really depended on my schedule. Then, I sent a link to the students for the signupgenius.com page. After the students had a few days to sign up, I pulled the tests according to the schedule and had them ready to go. I also made sure that my key was written and that I had two copies of it, one for me and one for the student. Once the students started showing up for their assigned times, I would give them one of the copies of the key to look at and I would go through the test and score it while they looked at the key. We would talk as I graded and I would give specific feedback on each problem and they would also ask questions about their test and about the material. The session really gave us a chance to talk about what their strengths and weaknesses were on the material, mistakes they made on the test, best approaches to fixing them, how they did on the home thinking that was assigned during the unit, as well as anything else that helped them in their journey through the chapter. Here is one session that I happened to jot down: Me: 'Hi. Are you ready?!?!?!' Student: 'I'm a bit nervous' Me: 'Well, I can understand, but everything's going to be ok!' <I start grading the first couple problems> Me: 'Oh, I like your method here for solving this. I haven't seen this used much.' Student: 'Thanks. It made more sense to me to do it that way. My friends don't like it, but I do.' <I grade another problem and the student loses all but 1 pt.> Student: 'I didn't really have time to study that one. I know you said to look at those types, but I just didn't have time' Me: 'That's understandable. It happens. No worries!' <We finish the grading of the test> Me: 'What do you think?' Student: 'I think I did really well. I'm a bit disappointed in myself on a couple of problems, but I like what I did on the one you mentioned.' Me: 'Well, don't be too hard on yourself - you have eight classes to juggle and mine is just one of eight! Here, let's look at your class grade when I enter your test score and home thinking score.' Student: 'That looks good. Thanks for taking the time to do this.' Me: 'You are welcome.' This is just one piece of dialogue, but you can see the power of sitting one on one with them - this is a conversation that I would never have had with them otherwise. When all students left the grading session, they would know their score on the exam, their home thinking score for the chapter, and their current grade in the class. First, the pros of the new system:
Second, the cons of the new system:
 Path Forward I used this method for the rest of the school year, except for the last test (because I had to turn the test around in only a few days so the scheduling method would not work). I will use it again this year as much as I can. The best part about it is the immediate feedback that it gives to the students, as well as the rapport that can be built between the student and the teacher. Next year, I think I will have the students do a reflection on the grading session, and I will also have a list of the chapter objectives at the grading session so that the students and I can talk about which objectives were met during the exam and which objectives still needed some effort. Many teachers on our staff began to use this system as well when time allowed and the feedback from them was mostly positive. Hopefully this will help you too! |
Archives
February 2021
Categories
All
AuthorJeff Watson is a Math teacher at the University Liggett School in Grosse Pointe Woods, MI. His work as a software engineer made him realize the need for problem solvers and critical thinkers in the workplace today. Jeff believes that the secondary math classroom should be a place of critical thinking, collaborative learning, and exploration which will cultivate the problem solvers and thinkers needed today. |





















































































 RSS Feed
RSS Feed
