Quadratics unit day 5: Using Cultures of Thinking to plan an entire unit.

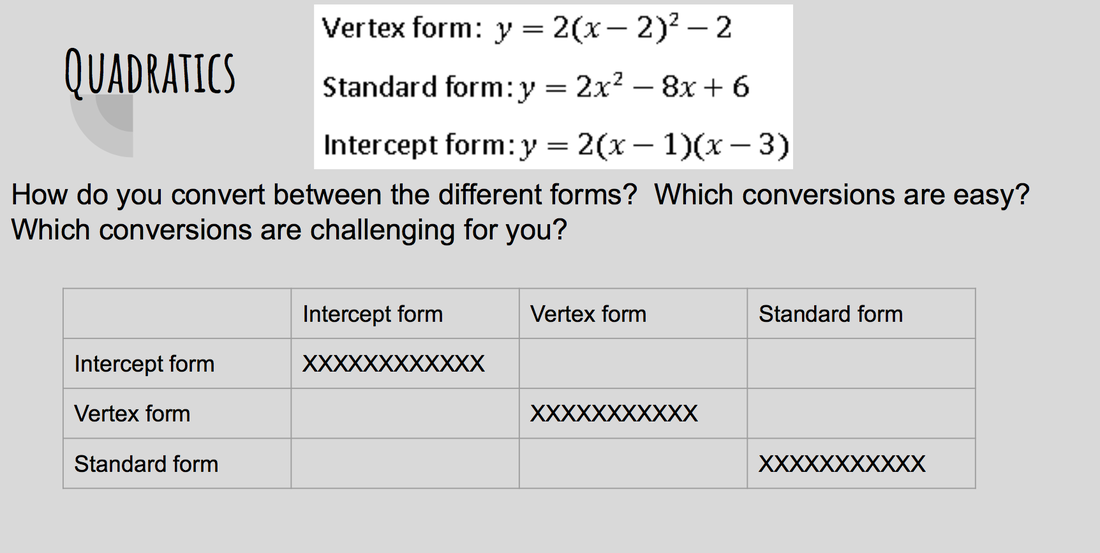
Before we embark on day 5, here is a quick summary of the first four days of the unit.  Click on each day if you would like to read the entire post:
Day 1: The students used Desmos polygraph to discuss the vocabulary surrounding the graphs of quadratics.
Day 2: We used the +1 Routine to generate all of our thinking about parabolas and quadratics.  
This led to the Desmos marble slides activity where the students wrote equations of quadratic functions in vertex form in order to generate graphs that 'catch' stars.
Day 3: The students continued the marble slides activity by plotting the vertex on each page of the activity, converting the equations from vertex form to standard form, and looking at the 'wideness' and 'narrowness' of parabolas.
​Day 4:  Students looked at three different forms of a quadratic: intercept form, standard form, and vertex form.  They had a discussion on what the benefits were of each form.  We then began to explore how to find the vertex using the standard formula (-b/(2a), -b^2/(4a)+c).  Students were having more trouble with this than expected as they were having trouble dealing with the negative signs in the formula.  They could easily self check their vertex as it was already plotted on their demos slides, but many of them were not getting matching answers.

For their home thinking that was due today, I had asked the students to manually find the vertex for each of the parabolas in the Desmos marble slides activity and to check their answers as they went along.  Each student logged in to their Demos marble slides activity and had it showing on their computer screens, with the home thinking that they created sitting out as well.  I used the Team Shake app to mix the class up in to pairs and gave them five minutes to discuss the results of their thinking.  I walked around and answered various questions about finding the vertex, looking at the math work as I went.  Some students were still having trouble simplifying correctly, while other students had mastered the concept.  I then had a discussion with the class about doing more practice with this concept.  It went something like this:
Me: As I was walking around, I noticed that some of you are still practicing and playing with this concept, while some of you seem to be getting the correct vertex every time.  If you are finding that you can't get the vertex every time, then you may need more practice.  Each of you has to decide where you are at and if you need more practice.
Student 1:  Mr. Watson, are there other practice problems that you can give us so we can find the vertex?
Me: I do have worksheets made up, but do you really need them?
Student 2: It would be nice to have.
Me: Let's suppose Moodle is down, though, and you can't get to the worksheet that is loaded on there.  What could you do instead?
Student 3:  We could generate a bunch of parabolas in desmos.com, like we did with the marble slides activity, and then try to find the vertex for each by hand.
Me: Sounds like you would have an infinite amount of examples to pick from, plus you would have the answers right there.
Student 4: Yeah it would be good to have the answers because that one time you forget to post them and I waited around and they never showed up.
<The class and I all laugh at this comment>
Me: Yeah, it's better to rely on yourself for the problems and answers than to wait for an old teacher like me <laughter here>

At this point I present the class with the following pictures of two different calculator screens:

I then pose this question to them: "Take a look at the calculator screens that I have put up on the overhead.  Take a minute and process what you are seeing here.  Think about what is happening on each calculator screen and be ready to discuss."  The students thought for several minutes.  I realized after we did this that it would have been a perfect moment to have the students do a 'See-Think-Wonder' routine; this is essentially what we did, though, but with different words.  I asked the students for some input and gave appropriate wait time.  The students chimed in and realized that what they were seeing was the calculation of the y coordinate of the vertex, done two different ways.    I then asked them which solution was 'better.'  This part surprised me, as most of the students chose the picture on the left.  Here were some of the comments:
"I would pick the one on the left because it makes more sense to me"
"The one on the left makes you think about what you are doing"
"The left one is easier to see what is going on"
"The one on the left gives you the steps, but the one on the right is quicker.  So, if you know what you are doing, do the one on the right; if you are still learning it do the one on the left."

For years, I didn't give the students a choice in the matter.  I made them calculate the y-coordinate using the screen on the right because I thought it was easier and cleaner; I didn't consider their viewpoint in that the steps made more sense to them if they entered it like the screen on the left.  I left this activity pleasantly surprised because their response to this was not at all what I had expected.  A student then asked, "Well, which one do you want us to do on the test?"  My reply was, "Well, can we ask that question but leave off the phrase 'on the test'?"  The student replied, "Well which one do you want us to do?"  I said, "Which one makes better sense to you?"  She said, "The one on the left for now, but maybe the one on the right."  My response was "well, do the one that make the most sense to you.  As mathematicians, there are many ways to do the same thing, and we sometimes need to do what we are comfortable with."

---

     I then showed the following slide to the class.  I gave them a minute to read it and then asked if they had questions on what they were expected to do.  One question that was asked was: "What do we put in each box in the chart?"  I said, "Each box is a process in and of itself.  Each box will most likely take up a good portion of a sheet of paper or a white board.  Feel free to use chart paper, loose leaf paper, a small white board, or one of the large white boards on the wall.  It is time to dig deep!  One recommendation: start with the vertex form to standard form cell, and then go from there."

     The next block of time, over 30 minutes, was one of organized chaos!  Students were all over the place in the classroom: some chose to work with pencil and paper, some used small white boards, some stood at the classroom white board, others were using the computer to look up items on the internet.  At one point, a group of students was standing around a laptop and when I went over they were watching a Khan academy video about completing the square.  I asked them what they were watching and they said that they had read that to convert from standard form to vertex form, they needed to complete the square, and they wanted to see what it was.  Now, I knew that completing the square was not a focus of this unit and it would be something that they would do in later years, but for now I did not want to extinguish their energy and excitement.  
     As I kept listening to the class, I heard things like 'foil', 'simplify', 'completing the square', and 'factoring.'  I was excited to hear these key words and phrases.  One pair of students were 'arguing' over one of the conversions because one of them entered it in wolframalpha.com and the other on symbolab.com and they got different answers.

One student came up to me and our conversations went something like this:
Student:  "Mr. Watson, I think I know how to convert from vertex form to standard form, but I'm not sure what to call it."  
Me: "well, what is it that you did to do this?"
Student: "I used foil and then made it simpler."  
Me: "well, that sounds like a good way to describe it - let's go with that and we can see what the class thinks later."  
Student: "We haven't spent any time on this, have we?"
Me: "Remember when you did the conversions in desmos last week?  And you checked them?"
Student: "OHHH!!!!  That's what we were doing when we were doing that.  It makes sense now!"

This made me laugh as this particular student finally made the connection of something we were doing a while back.  This type of thing used to irritate me before, but now I accept that learning is a process and different students process and master things at different rates.

Class was nearing the end so I asked the students to pick two conversions that they felt the most comfortable with at this point in time.  I asked them to find two quadratic equations and convert them accordingly for our next class.

Please stay tuned for Quadratics Day 6, where we will fill in the conversion chart, which will naturally lead us to foil'ing as well as factoring!